【ベストコレクション】 reflection over y=x axis 144254-Reflection over y=x axis rule
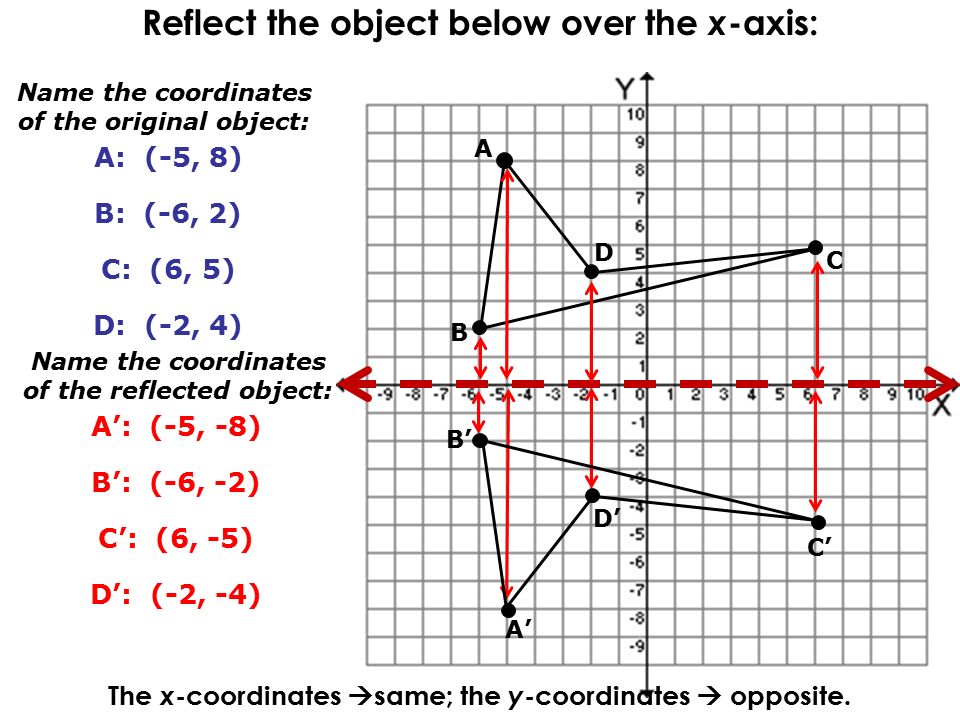
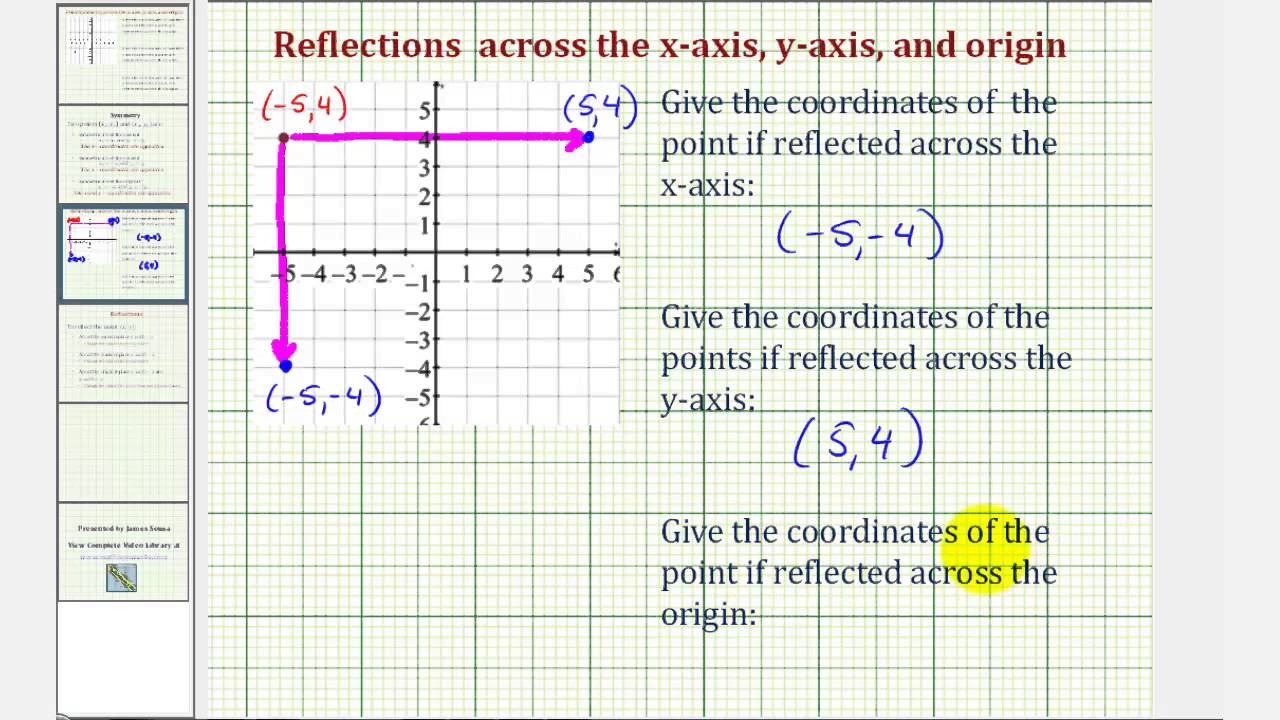
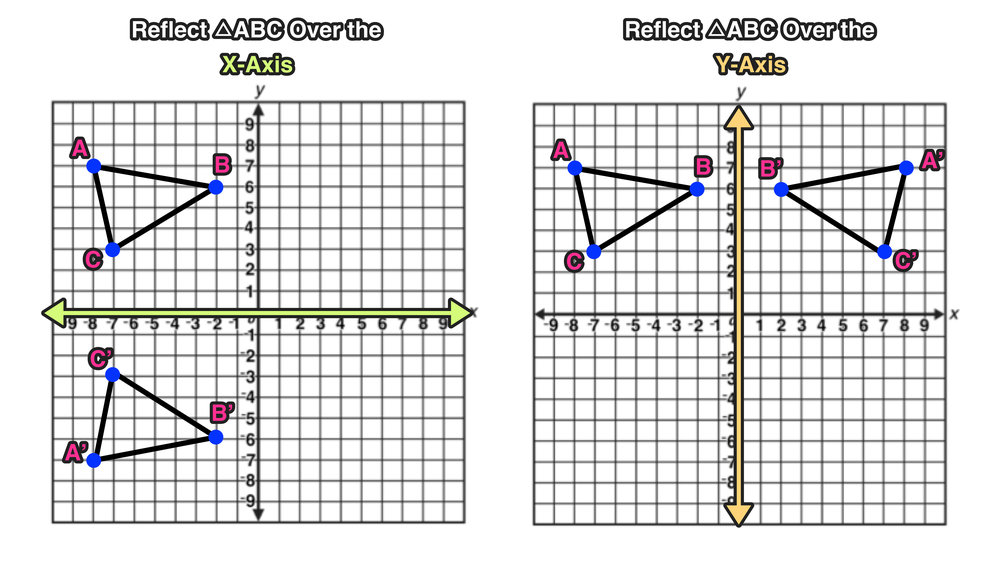
The reflection transformation may be in reference to X and Yaxis Reflection over Xaxis When a point is reflected across the Xaxis, the xcoordinates remain the same But the Ycoordinates are transformed into its opposite signs Therefore, the reflection of the point (x, y) across Xaxis is (x, y) Reflection over YaxisThis is a KS3 lesson on reflecting a shape in the line y = −x using Cartesian coordinates It is for students from Year 7 who are preparing for GCSE This page includes a lesson covering 'how to reflect a shape in the line y = −x using Cartesian coordinates' as well as a 15question worksheet, which is printable, editable and sendableA reflection across xaxis is nothing but folding or flipping an object over the x axis The original object is called the preimage, and the reflection is called the image If the preimage is labeled as ABC, then t he image is labeled using a prime symbol, such as A'B'C' An object and its reflection have the same shape and size, but the figures face in opposite directions
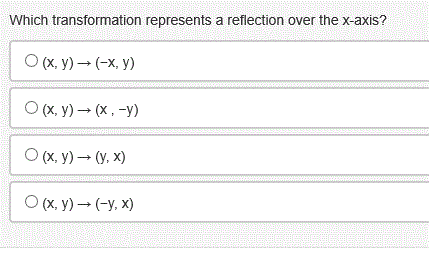
Which Transformation Represents A Reflection Over The Chegg Com
Reflection over y=x axis rule
Reflection over y=x axis rule-Note Reflecting a figure over the yaxis can be a little tricky, unless you have a plan In this tutorial, see how to use the graph of a figure to perform the reflection When reflecting over (across) the xaxis, we keep x the same, but make y negative Reflection Across the XAxis Reflection Over Y Axis When reflecting over (across) the yaxis, we keep y the same, but make xnegative



Reflect Function About Y Axis F X Expii
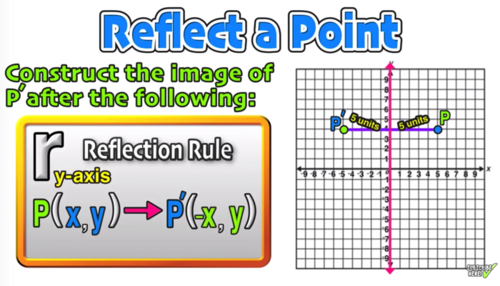
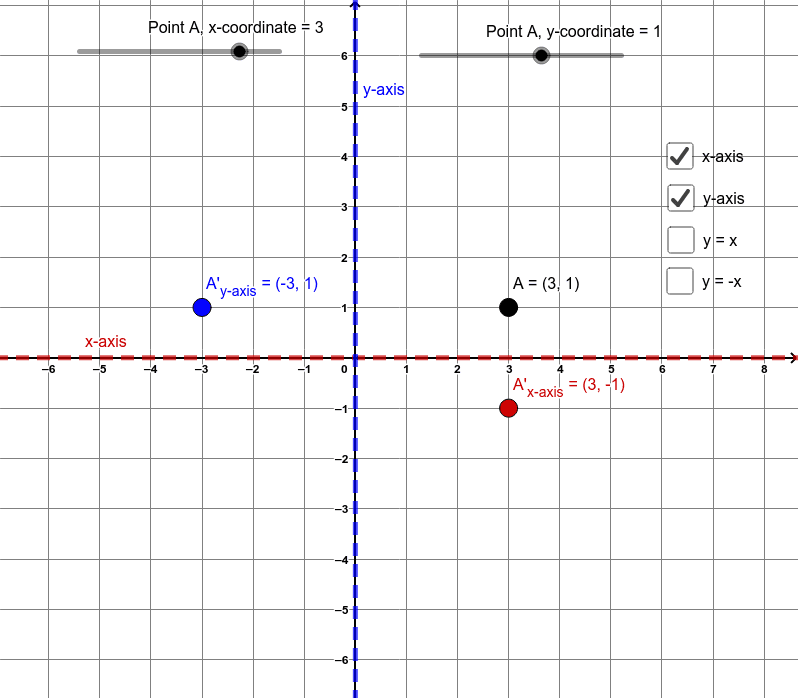
Determine the line of reflection The most common lines of reflection are the xaxis, the yaxis, or the lines y = x or y=−x The preimage has been reflected across he yaxis This means, all of the xcoordinates have been multiplied by 1 You can describe the reflection in words, or with the following notation ry−axis(x,y)→(−x,y)A reflection of a point over the y axis is shown The rule for a reflection over the y axis is (x, y) → (− x, y) Reflection in the line y = x A reflection of a point over the line y = x is shown A reflection over the yaxis leaves the point the same distance above the xaxis (that is the ycoordinate value does not change) The xcoordinate value becomes an equal distance from the yaxis but on the other side of the yaxis (that is the xcoordinate value becomes the negative of what it was)
In a reflection transformation, all the points of an object are reflected or flipped on a line called the axis of reflection or line of reflection Example A reflection is defined by the axis of symmetry or mirror lineIn the above diagram, the mirror line is x = 3 A) reflect over the yaxis, reflect over the xaxis, rotate 180° B) rotate 180°, reflect over the xaxis, reflect over the line y=x C) reflect over the xaxis, rotate 180°, reflect over the xaxisQ Reflect the point over the yaxis What is the coordinate of its image?
Which statement accurately describes how to reflect point A (3, −1) over the yaxis? Welcome to The Reflection of 3 Vertices Over the x or y Axis (A) Math Worksheet from the Geometry Worksheets Page at MathDrillscom This math worksheet was created on and has been viewed 32 times this week and 105 times this month It may be printed, downloaded or saved and used in your classroom, home school, or other educationalGraphing Reflections of latexf\left(x\right)={\mathrm{log}}_{b}\left(x\right)/latex When the parent function latexf\left(x\right)={\mathrm{log}}_{b}\left(x\right)/latex is multiplied by –1, the result is a reflection about the xaxis When the input is multiplied by –1, the result is a reflection about the yaxis




Learn About Reflection Over The Line Y X Caddell Prep Online



1
A reflection (or flip) is one kind of transformation The reflection of a point is another point on the other side of a line of symmetry Both the point and its reflection are the same distance from the line The following diagram show the coordinate rules for reflection over the xaxis, yaxis, the line y = x and the line y = x Scroll downQ Reflect over xaxis Which point would be at (3, 6) Q Which axis is the horizontal (leftright) one?Graph functions using reflections about the xaxis and the yaxis Another transformation that can be applied to a function is a reflection over the x – or y axis A vertical reflection reflects a graph vertically across the x axis, while a horizontal reflection reflects a graph horizontally across the y axis
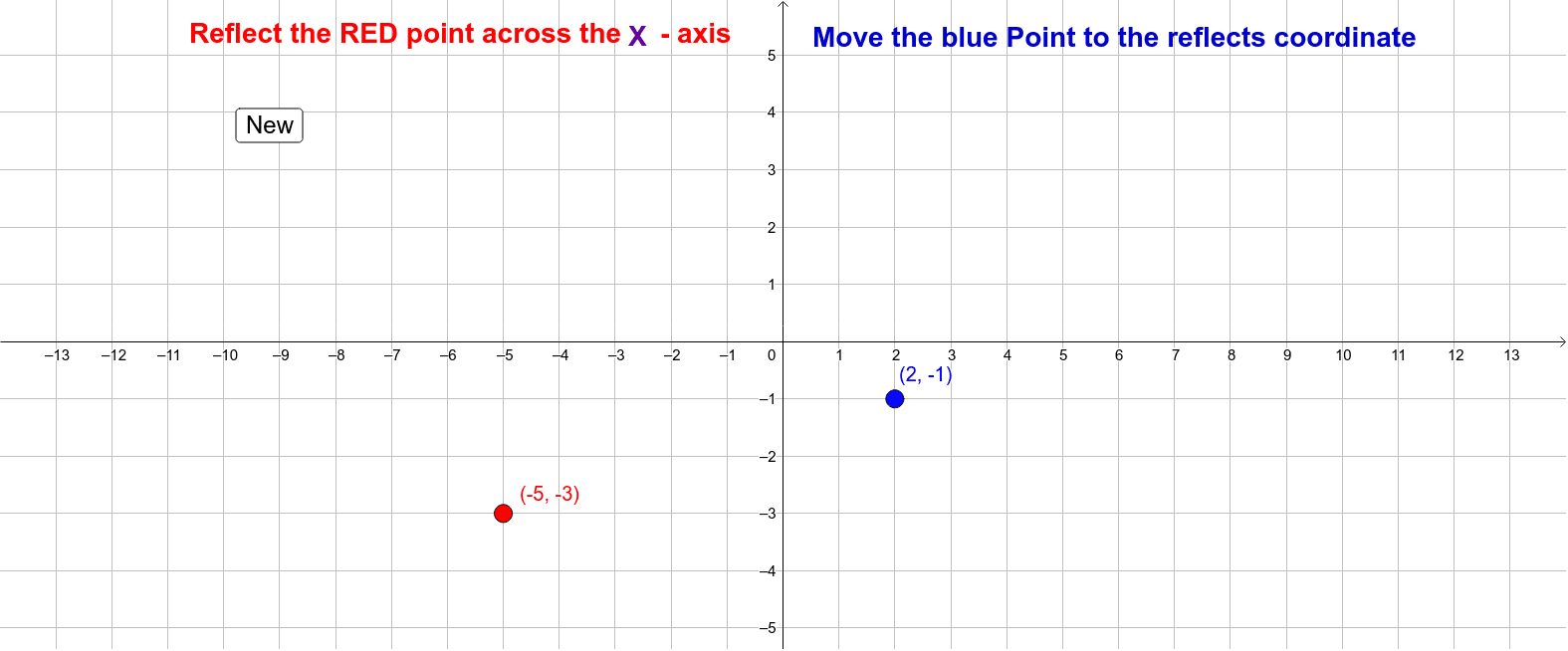



Reflection Point 2 Geogebra




How To Reflect A Graph Through The X Axis Y Axis Or Origin
The best way to practice drawing reflections over y axis is to do an example problem Example Given the graph of y = f (x) y=f(x) y = f (x) as shown, sketch y = f (− x) y = f(x) y = f (− x) Remember, the only step we have to do before plotting the f(x) reflection is simply divide the xcoordinates of easytodetermine points on ourReflection about the yaxis Reflection about the line y = x Once students understand the rules which they have to apply for reflection transformation, they can easily make reflection transformation of a figure Let us consider the following example to have better understanding of reflectionConstruct a line from A perpendicular to the yaxis, determine the distance from A to the yaxis along this perpendicular line, find a new point on the other side of the yaxis that is equidistant from the yaxis
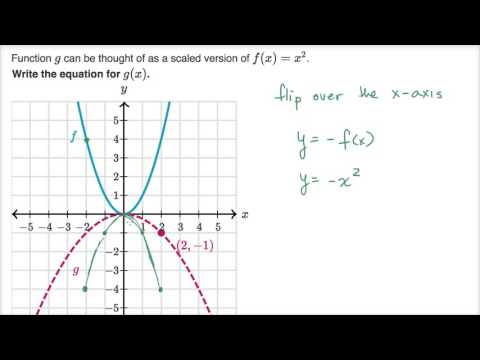



Scaling Reflecting Parabolas Video Khan Academy




9 13 18 Reflections Geometry Blog
33 $100 Zip There are 3 mazes on reflecting a point over the xaxis and yaxis, one without using a graph, and a set of 12 problems on reflections over y = 1, x = 3This product is included in the Transformation MAZE BUNDLE for $450Posted 4/24/16 so 50% off through 4/Reflection over some axis is a common mathematical transformation Generally, reflection is accomplished by changing the sign of something Suppose weRelated Pages Properties Of Reflection Transformation More Lessons On Geometry What is Reflection?




Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation



Ppt 9 1 Reflections Powerpoint Presentation Free Download Id
Unlock StepbyStep reflect across y=2x Extended Keyboard ExamplesFor triangle ABC with coordinate points A (3,3), B (2,1), and C (6,2), apply a reflection over the line y=x By following the notation, we would swap the xvalue and the yvalue A (3,3), B (2,1), and C (6,2) would turn into A' (3,3), B' (1,2), and C' (2,6)Geometry reflection A reflection is a "flip" of an object over a line Let's look at two very common reflections a horizontal reflection and a vertical reflection



How To Reflect A Graph Through The X Axis Studypug




Reflection Mathbitsnotebook A1 Ccss Math
Systems of Linear EquationsAlex Phipps Ch6 Geogebra Project;23 Questions Show answers Q Flipping a figures is a Q Is the picture being reflected in the yaxis or xaxis?



Assignment 2 Transforming Parabolas



Reflecting Over The X Axis And Y Axis Ppt Video Online Download
Reflection in yaxis (green) f(−x) = −x 3 − 3x 2 − x − 2 Even and Odd Functions We really should mention even and odd functions before leaving this topic For each of my examples above, the reflections in either the x or yaxis produced a graph that was different But sometimes, the reflection is the same as the original graph Reflection over the xaxis is a type of linear transformation that flips a shape or graph over the xaxis Every point above the xaxis is reflected to its corresponding position below the xaxis; The rule for reflecting over the X axis is to negate the value of the ycoordinate of each point, but leave the xvalue the same Click to see full answer Likewise, what is the rule for a reflection across the X axis?



Reflection Over The X And Y Axis The Complete Guide Mashup Math




Reflection Over The Y X Line Youtube
We can remember the reflections by y=f (x) is a reflection acroos the xaxis because the negative is in the first term of the right hand side of the equation (f) and because the first coordinate we plot in a graph is the Xcoordinate Reflection in a Line A reflection over a line k (notation r k) is a transformation in which each point of the original figure (preimage) has an image that is the same distance from the line of reflection as the original point but is on the opposite side of the line Remember that a reflection is a flip Under a reflection, the figure does not change size 👉 Learn how to reflect points and a figure over a line of symmetry Sometimes the line of symmetry will be a random line or it can be represented by the x
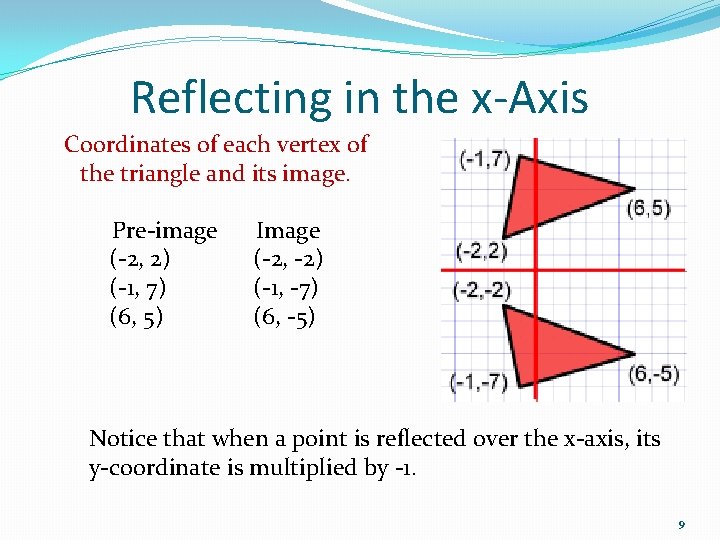



Reflections 1 Reallife Reflections Animation Architecture Graphic Design




Answered Rules For Reflections On A Coordinate Bartleby
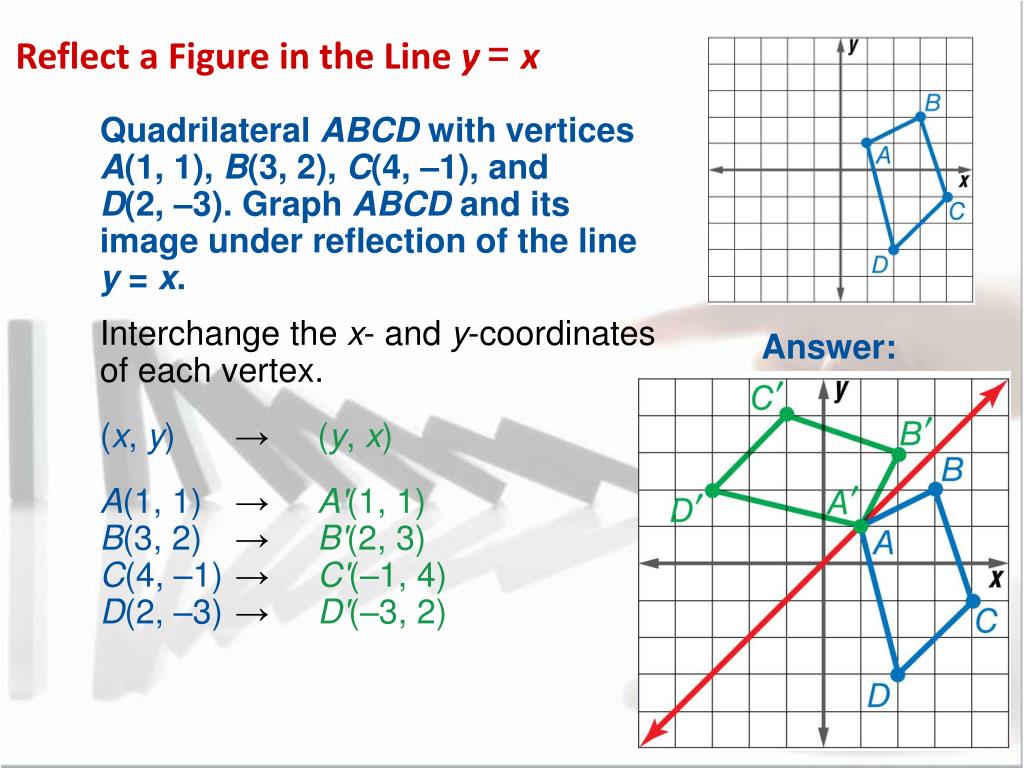
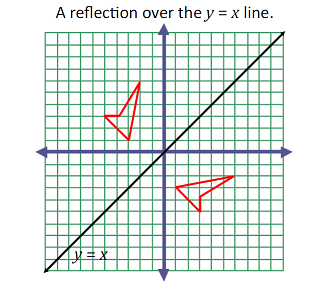
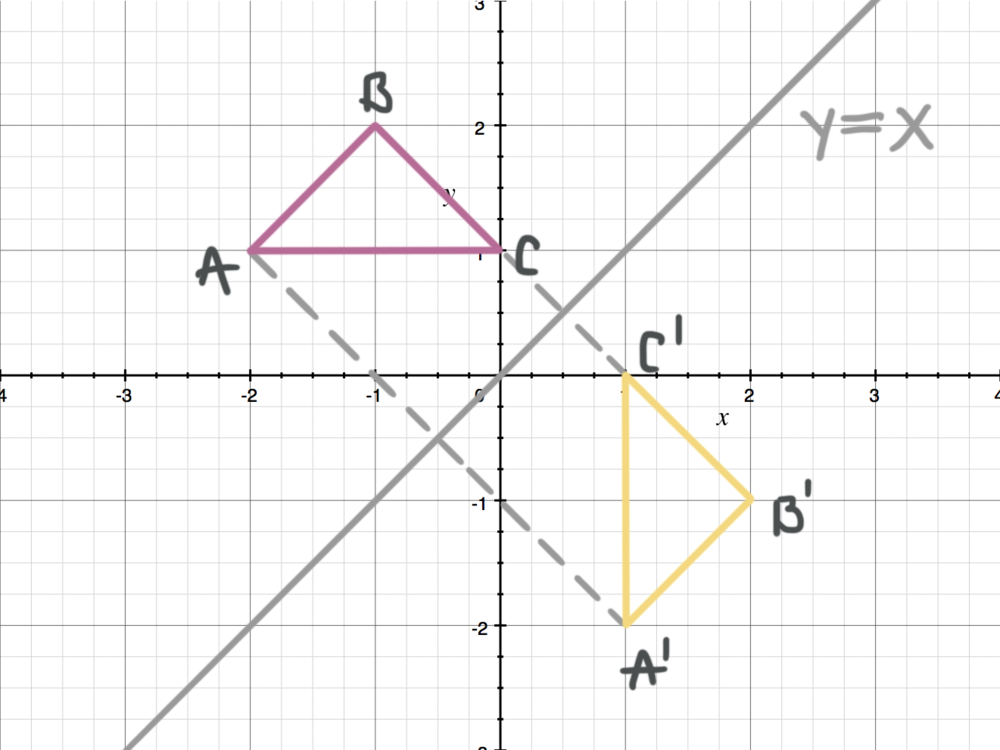
Geometry 3 people liked this ShowMe Flag ShowMe Viewed after searching for reflect over x= 1 reflection over the line y=x Reflection over y=x reflection over yaxis reflectionY X W I 3) reflection across the yaxis x y B S Z 4) reflection across the xaxis x y T R I 5) reflection across the yaxis x y M P Z ©j p2D0j1L5t lKVuJtqaD zSeo^fNtuwpalrYei ELdLfCCd n vAOlklA AroiKgLhwtHsj YrqeBsJelrmvPefR Y KMzaHd_eC wwviFtZhF dIJnmfHiAnfiGtJeX nGpeSo_mAeItXrHyx The Line Y=X Another common reflection occurs when a preimage is reflected over the line y = x Examine the drawing below to see the relationship between the coordinates of the preimage and image Notice that the xand yvalues are reversed In otherwords, the xvalue of the preimage maps onto the yvalue of the image and vice versa




Ppt Reflect Over Y X Powerpoint Presentation Free Download Id




Picture Of Reflection Across Y Axis Reflection Math Reflection Math
Start studying reflection over x and y axis Learn vocabulary, terms, and more with flashcards, games, and other study toolsReview how to reflect objects across the x and y axis on the coordinate plane by following simple rulesThis lesson is given by Taina MaisonetYou can followReflections and Rotations We can also reflect the graph of a function over the xaxis (y = 0), the yaxis(x = 0), or the line y = x Making the output negative reflects the graph over the xaxis, or the line y = 0 Here are the graphs of y = f (x) and y = f (x)



How To Reflect A Graph Through The X Axis Studypug
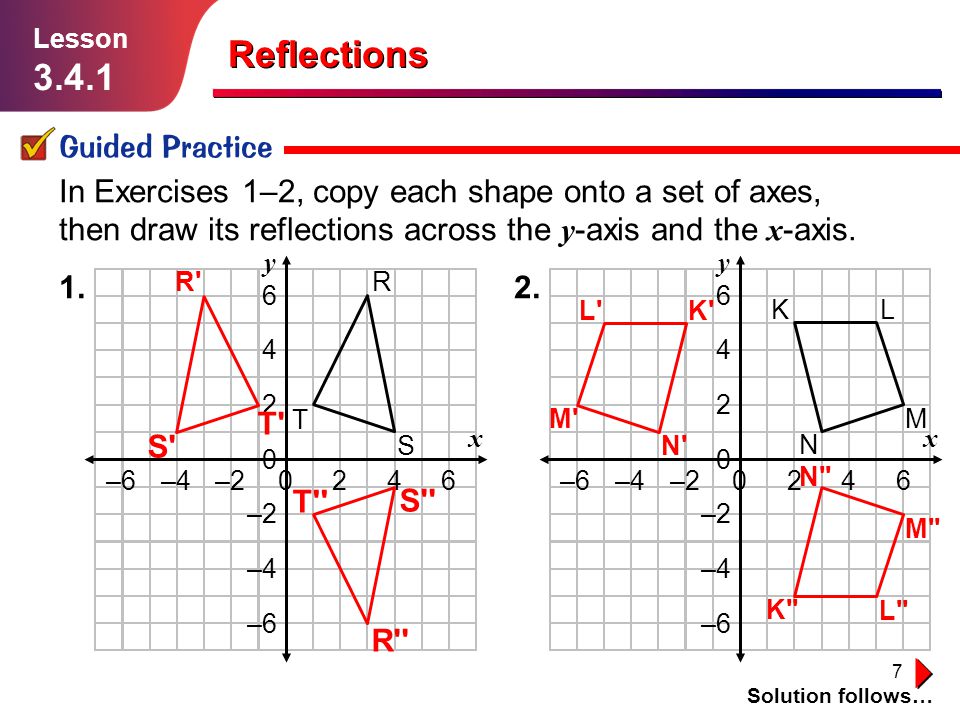



Reflections Lesson Ppt Video Online Download
Reflection across the xaxis y = − f ( x) y = f (x) y = − f ( x) The concept behind the reflections about the xaxis is basically the same as the reflections about the yaxis The only difference is that, rather than the yaxis, the points are reflected from above the xaxis to below the xaxis, and vice versa Basic ConceptsGet the free "Reflection Calculator MyALevelMathsTutor" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Education widgets in WolframAlphaWhen you reflect a point across the line y = x, the xcoordinate and ycoordinate change places




How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com




How To Find A Reflection Image
On the screen you can see that the graph of this equation is a parabola The reflection of such a parabola over the x axis is simply written as y = (x ^2) In other words, the function of f (x)Reflection over xaxis (line of reflection x axis) Reflectionflip in which image has opposite orientation Reflection over line y=x or y=Reflection over line y=x or y=xx Reflection over y=x Point (x,y) reflects to point (y,x) Reflection over line y=x Point (x,y) reflects to point (y,x) Title ReflectionsWhen you reflect over xaxis the coordinates are (x,y) and when you reflect over the yaxis the coordinates are (x,y If you want to reflect over y=x then the coordinates are (y,x) If you want to reflect over y=x the coordinates are (y,x) Comment
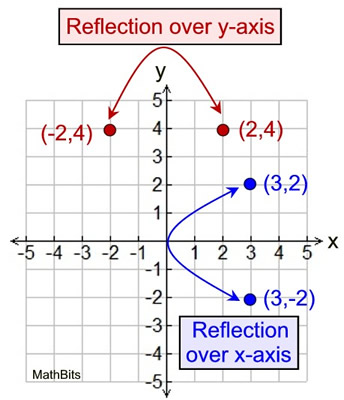



Graphing Coordinate Axes Mathbitsnotebook Jr




Ixl Reflections Over The X And Y Axes Graph The Image 7th Grade Math
A reflection flips a figure over a line to create a mirror image When a point is reflected over the x axis, the y coordinate changes signJust select an axis from the dropdown and enter the coordinates, the point reflection calculator will show the result Code to add this calci to your website The above point reflection calculator is capable of giving point reflection for your coordinates based on the Xaxis, Yaxis, and originEvery point below the xaxis is reflected to its corresponding position above the xaxis Contents Reflection over the xaxis for Sets of Coordinates (x, y),
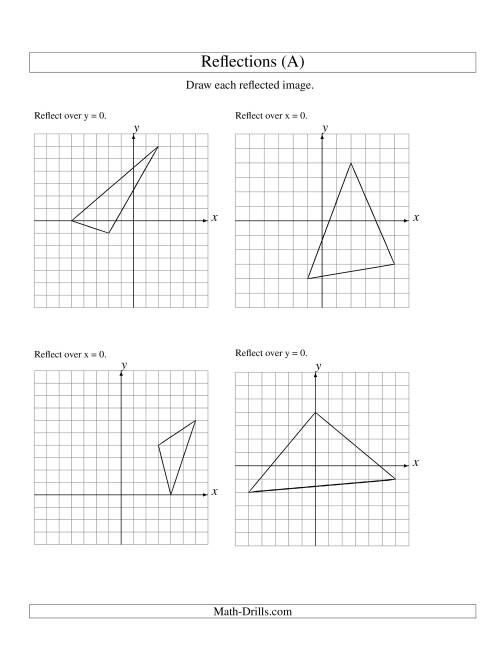



Reflection Of 3 Vertices Over The X Or Y Axis A




Picture Of Reflection In The Line Y X Reflection Math Math Reflection
You can also negate the value depending on the line of reflection where the xvalue is negated if the reflection is over the yaxis and the yvalue is negated if the reflection is over the xaxis Either way, the answer is the same thingSo, if the point of reflection is labelled as X, Y then the same coordinates across the XAxis would be X, Y The Reflection upon the YAxis As the contrary case of XAxis, the YAxis here will stay the same while the Xcoordinates transform with their opposite symbols when the reflection takes place across the YAxis To reflect it over the yaxis, the reflection will be in quadrant 2, still above the xaxis, but now left of the yaxis The distance the reflection is from the yaxis is the same as the original point is from the yaxis The reflection of (5, 5) over the yaxis is (5, 5) To reflect any point over the yaxis, replace the xcoordinate with the



1
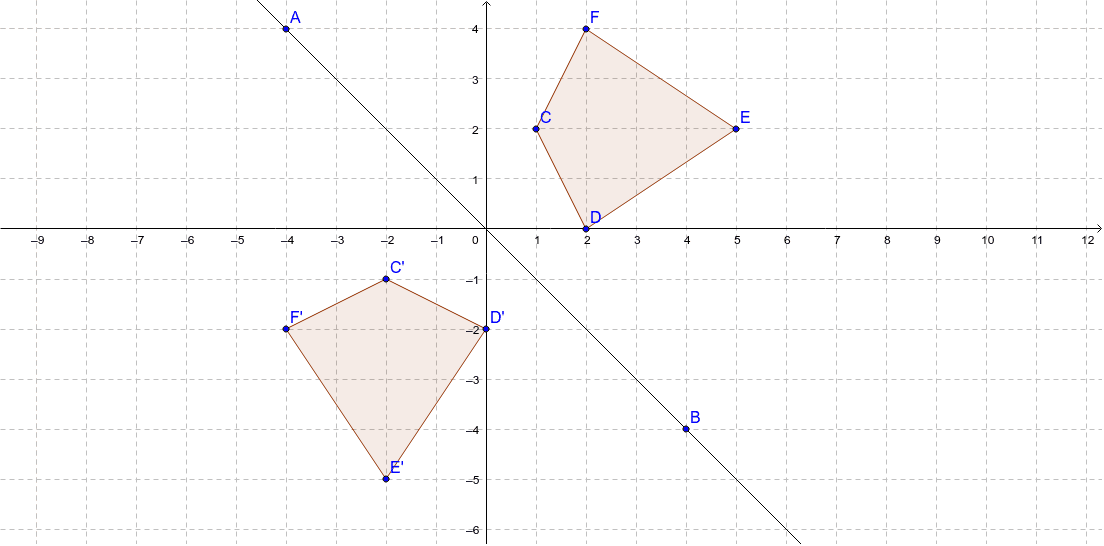



Reflection Over Y X Geogebra
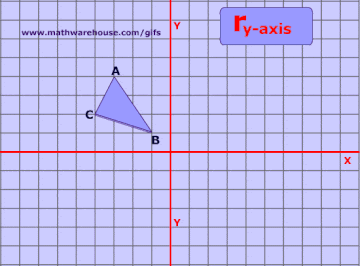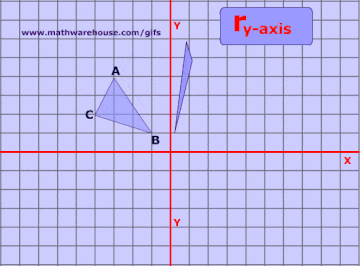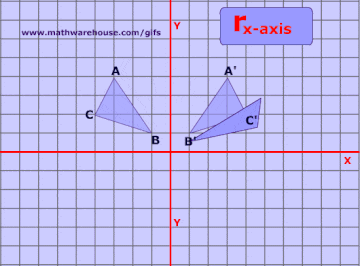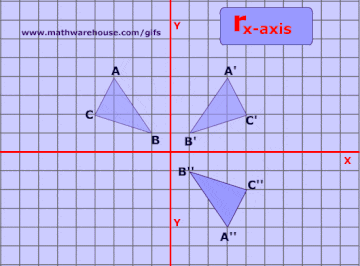
Reflection over the yaxis Discover Resources Practice2;Reflection over the yaxis A reflection in the yaxis can be seen in diagram 4, in which A is reflected to its image A' The general rule for a reflection over the yaxis $ r_{yaxis} \\ (A,B) \rightarrow (A, B) $




Reflect Function About Y Axis F X Expii



What Does It Mean To Reflect Over The Y X Line Quora



Q Tbn And9gctvjthqkdwbpqfxmfukfqyrhm8wtlbtfnfu2u0osqggs0pct8ms Usqp Cau




A Line Segment Goes From 1 2 To 4 1 The Line Segment Is Reflected Across X 1 Reflected Across Y 3 And Then Dilated About 2 2 By A Factor




Which Transformation Represents A Reflection Over The Chegg Com



Solution If A 3 5 Is Reflected Over The Y Axis What Are The New Coordinates Of A




Reflection Mathbitsnotebook A1 Ccss Math
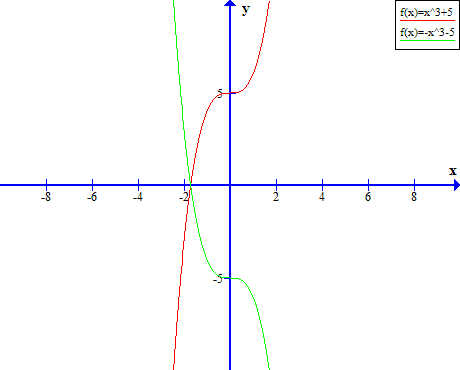



If The Graph Y X 3 5 Is Reflected In The X Axis What Is The New Equation Socratic
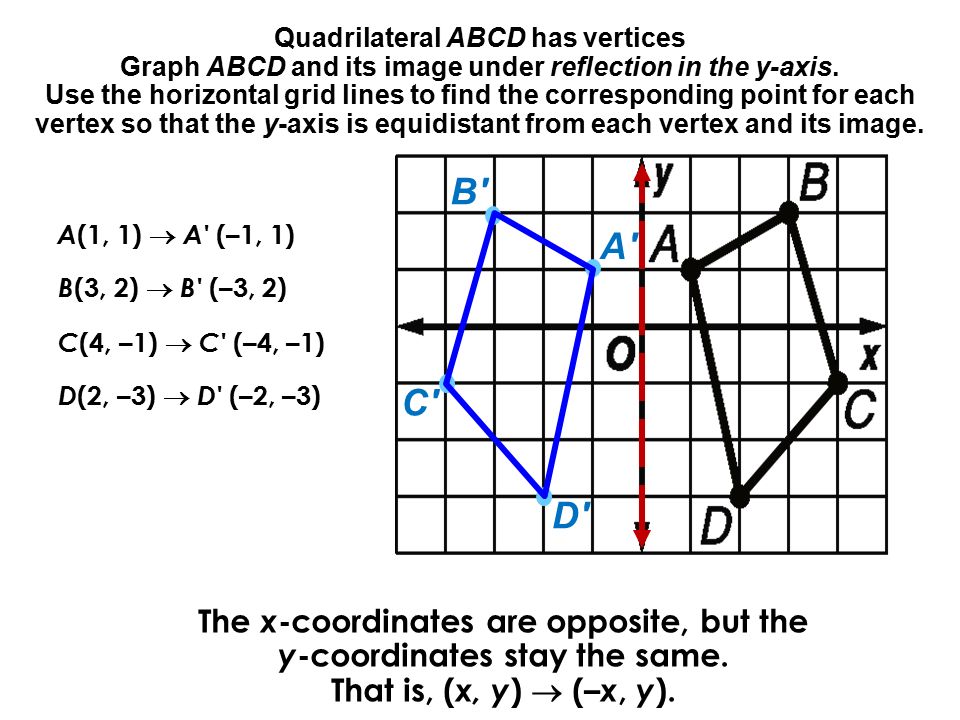



Reflecting Over The X Axis And Y Axis Ppt Video Online Download




Reflection Definition Reflection In The Coordinate Plane
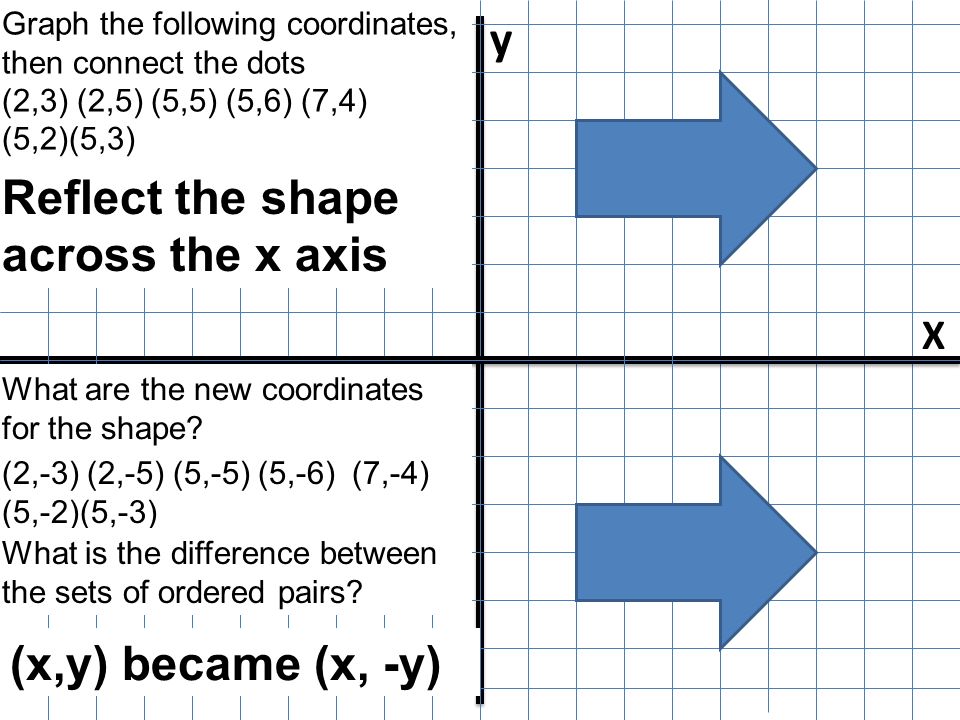



Reflections Reflect The Shape Across The X Axis Graph The Following Coordinates Then Connect The Dots 2 3 2 5 5 5 5 6 7 4 5 2 5 3 What Ppt Download




Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation




Graph Functions Using Reflections About The X Axis And The Y Axis College Algebra




Reflection Rules How To W 25 Step By Step Examples
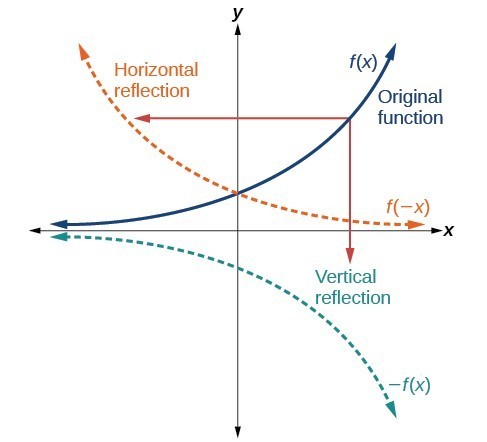



Graph Functions Using Reflections About The X Axis And The Y Axis College Algebra




Transformation Reflection Over The Line Y X Youtube
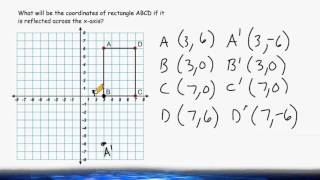



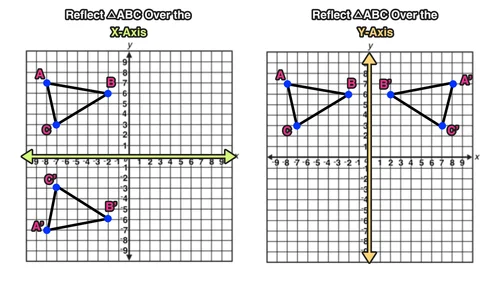
Transformations Reflection Across The X Axis Youtube
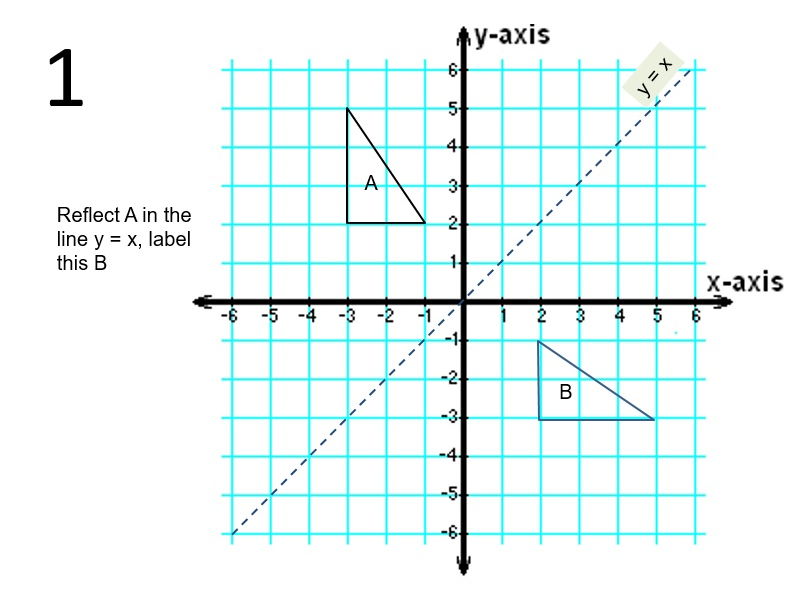



Diagonal Reflections In The Line Y X Teaching Resources



Http Www Showmethemath Com Nates notes on math Nates notes on geometry Nates notes on transformations Thefivebasicreflectionsintheplane Pdf




Reflection Over The X And Y Axis The Complete Guide Mashup Math




Reflect The Square Across The Line Y X Followed By Chegg Com
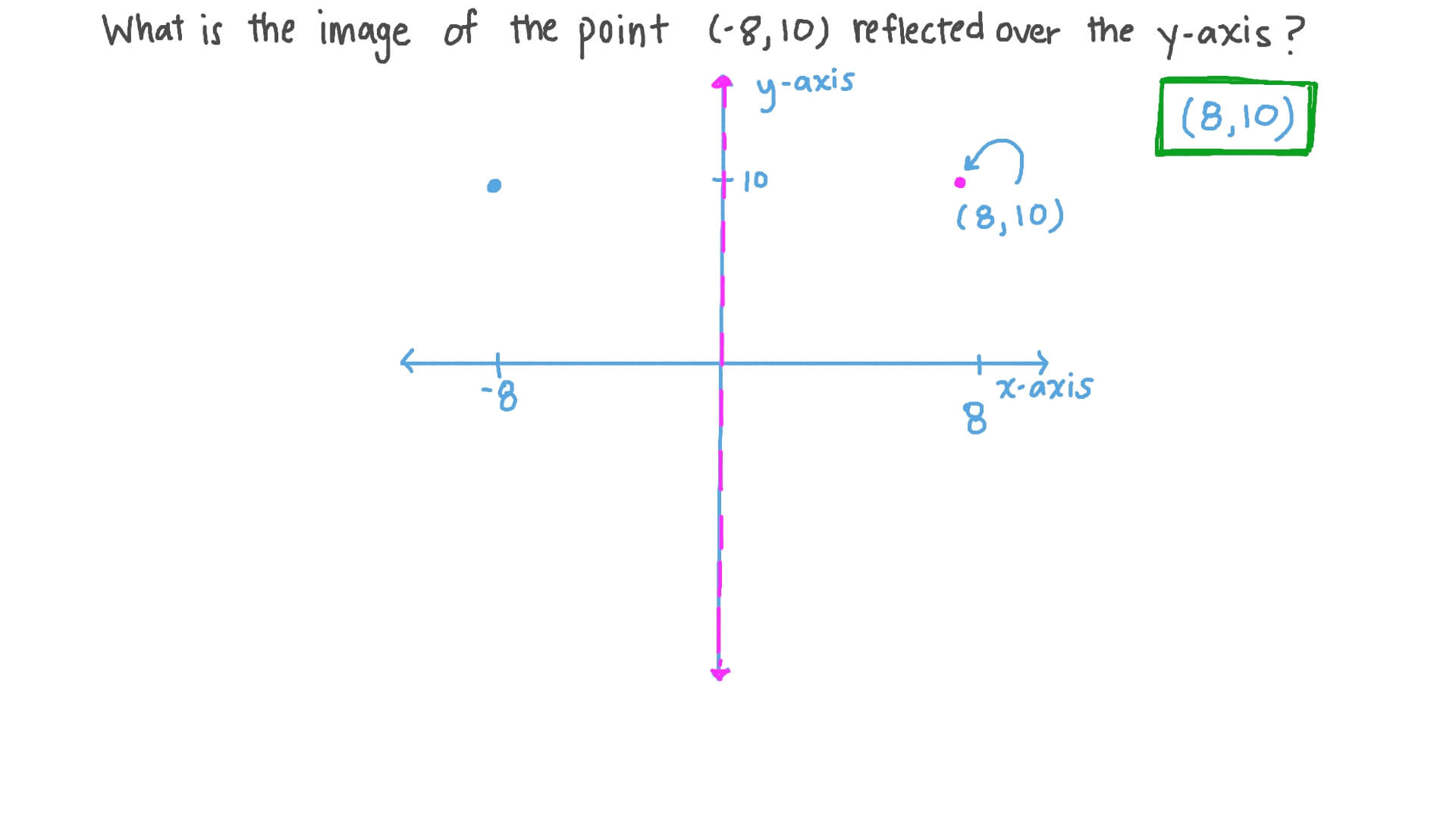



Question Video Determining The Coordinates Of A Point After Reflection Nagwa




How To Reflect Quadratic Equations Video Lesson Transcript Study Com



Reflection Over A Line Expii
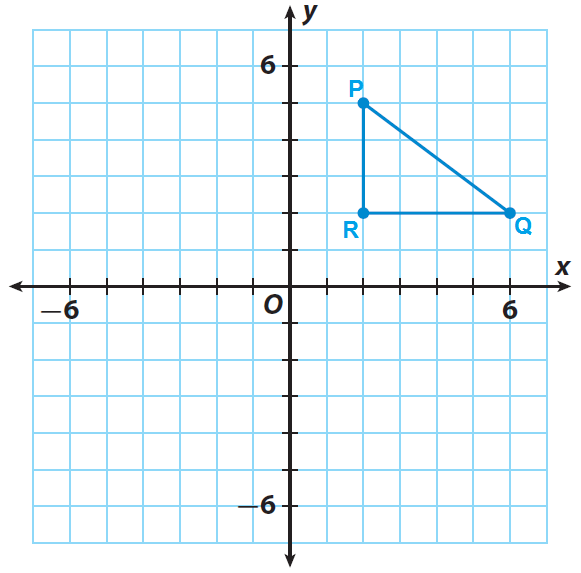



Properties Of Reflections




Reflecting Shapes Article Reflections Khan Academy
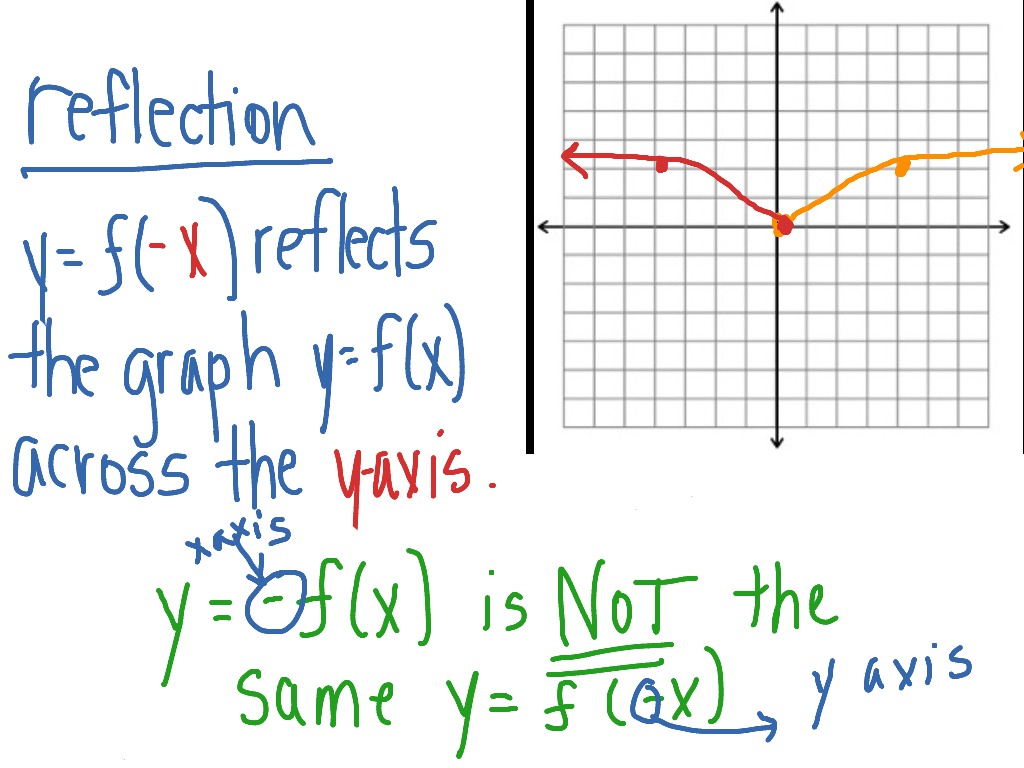



Reflection Across The Y Axis Math Functions Showme




Reflection



Great Minds Eureka Math Blog




Reflection Over The X And Y Axis The Complete Guide Mashup Math




Reflection Mathbitsnotebook A1 Ccss Math



Reflection Over The X And Y Axis The Complete Guide Mashup Math




Reflecting Points Video Reflections Khan Academy




Reflection Mathbitsnotebook A1 Ccss Math



Search Q Coordinate Plane Tbm Isch



Reflections Geometry Abroad




Reflection Transformation Matrix



How To Reflect A Graph Through The X Axis Studypug




Reflect Function About Y Axis F X Expii




Reflecting A Shape In The X Axis Using Cartesian Coordinates Key Stage 3



Ex Reflect A Point About The X Axis Y Axis And The Origin Youtube




Reflection Over The Line Y X Math Showme



Reflecting Figures In Coordinate Space Krista King Math Online Math Tutor




12 Reflections Pdf




Ixl Reflections Over The X And Y Axes Graph The Image 8th Grade Math




Reflect The Square Across The X Axis Followed By A Chegg Com




Reflection Transformation Matrix




How To Reflect A Graph Through The X Axis Y Axis Or Origin



Reflection Rules Geogebra




What Are The Coordinates Of Point A 4 1 After It Has Been Reflected Over The Y Axis Socratic




Ixl Reflections Find The Coordinates Grade 7 Maths Practice




Reflection Transformation




Reflection In Geometry Examples Solutions Videos Worksheets Games Activities



Reflection Across The X Axis Reflection Across The Chegg Com




Reflection Transformation



Illustrative Mathematics




Reflection Mathbitsnotebook A1 Ccss Math




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines




Reflection Rules How To W 25 Step By Step Examples




A Translation X Y X 5 Y Reflection Across Y Axis B Translation X Y X Y 5 Brainly Com



Reflection Over The X And Y Axis The Complete Guide Mashup Math
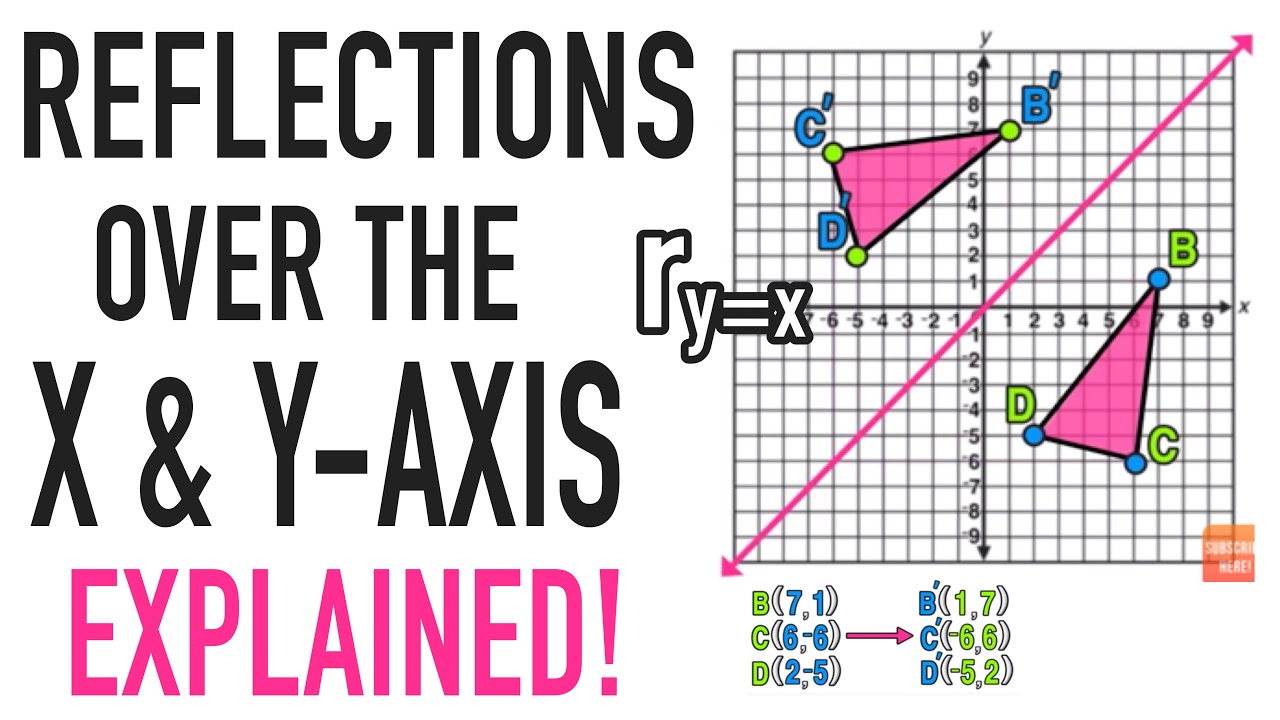



Reflections Over The X Axis And Y Axis Explained Youtube




Reflections How To Reflect A Point Reflect Point Across X Axis Y Axis And Other Lines Reflection Math Maths Activities Middle School Fun Math Worksheets




4 4 Geometric Transformations With Matrices Objectives To




Rectangle Abcd Is Reflected Over The X Axis What Rule Shows The Input And Output Of The Reflection Brainly Com
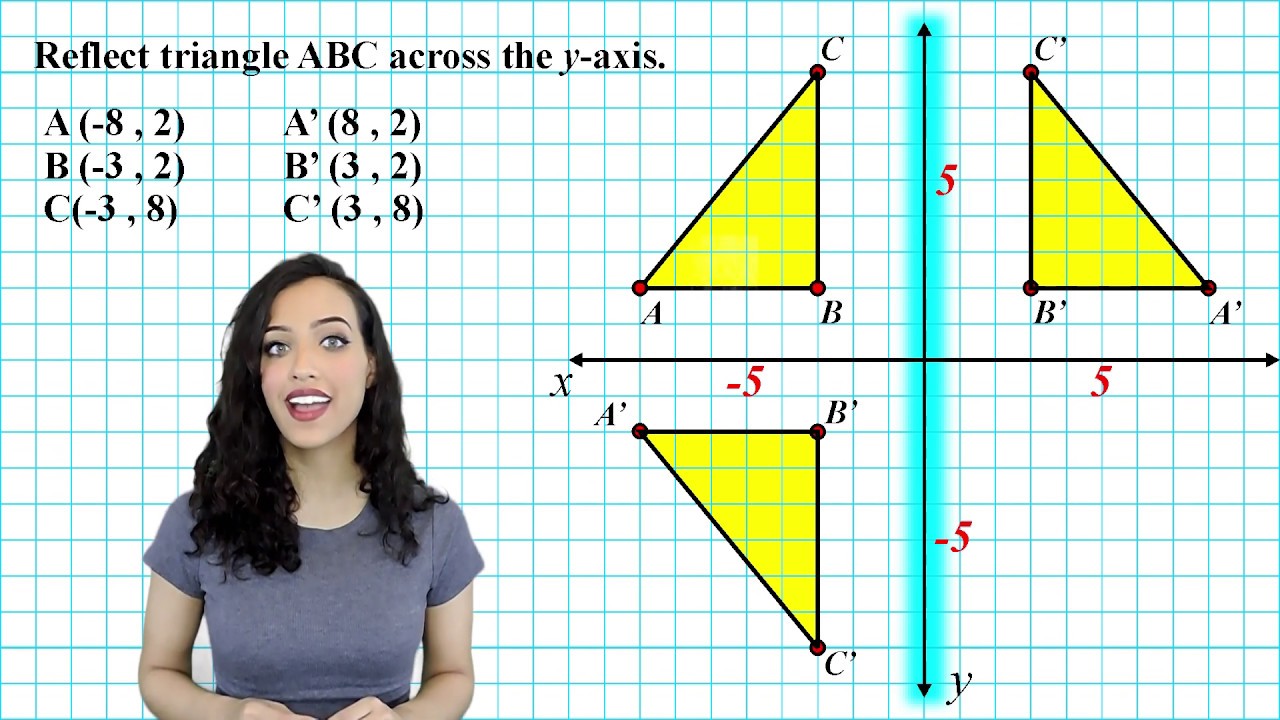



Reflection Across The X And Y Axis Youtube
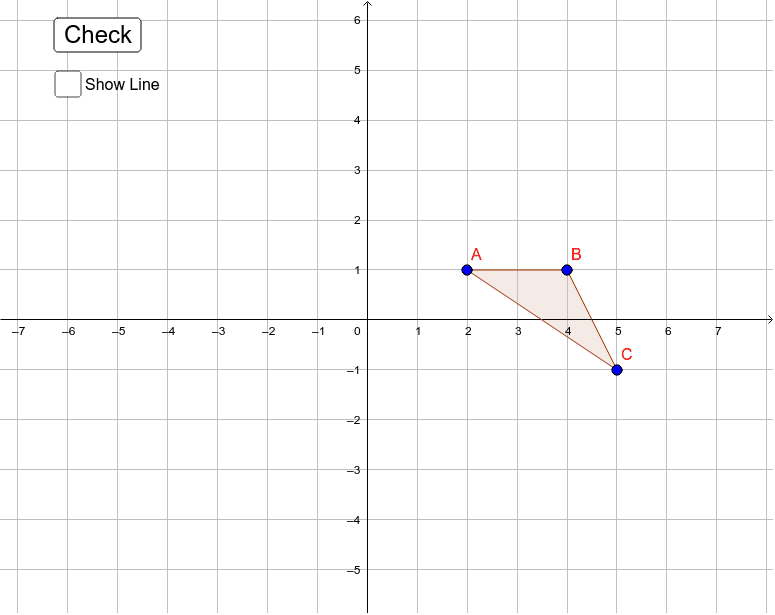



Reflection Over Y X Geogebra
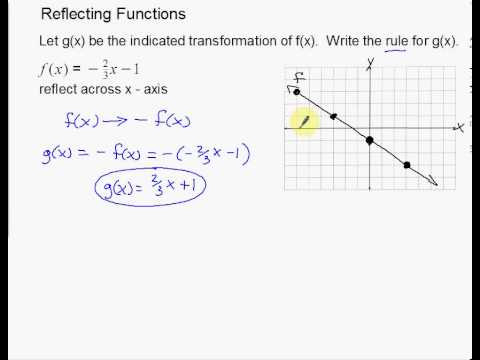



Linear Reflections Across X And Y Axis Example Youtube



Biomath Transformation Of Graphs




Reflections Through The Axes And The Lines Y X And Y X Geogebra
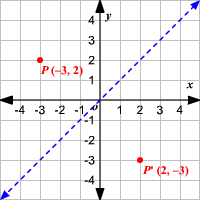



Reflections
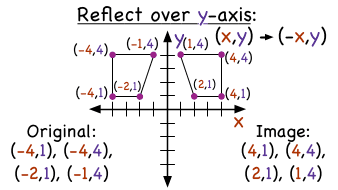



How Do You Use Coordinates To Reflect A Figure Over The Y Axis Virtual Nerd



Transformation Reflection Over X Axis
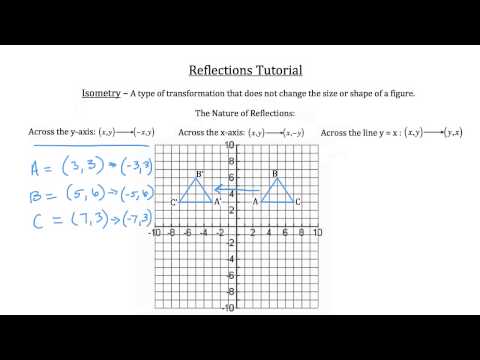



Reflection Notes Videos Qa And Tests Grade 9 Optional Mathematics Transformation Kullabs



Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines



Reflections Math Analysis Functions



コメント
コメントを投稿